The relationship expressed in the form of a mathematical function defines a situation for a given set of conditions and properties. Since we allowed the independent dimension to change, it was no longer a fixed quantity but became a variable. The graph of the function reflected a horizontal change of the independent variable with a vertical change in the dependent dimension. This graph allows us to visualize how a situation changes with respect to a change in the conditions. This chapter will focus on analyzing graphs and how they represent change.
To find out how much the function, 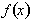 , changes between two points
, changes between two points 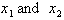 , we simply enter in the two values for the independent variable x and then calculate the difference between the dependent variable, f, for those given conditions. Remember that a variable is nothing more than a dimension that is allowed to change or take on any value. Thus, from
, we simply enter in the two values for the independent variable x and then calculate the difference between the dependent variable, f, for those given conditions. Remember that a variable is nothing more than a dimension that is allowed to change or take on any value. Thus, from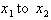 , the change in the independent variable , referred to as
, the change in the independent variable , referred to as 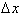 is:
is:
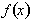 , changes between two points
, changes between two points 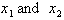 , we simply enter in the two values for the independent variable x and then calculate the difference between the dependent variable, f, for those given conditions. Remember that a variable is nothing more than a dimension that is allowed to change or take on any value. Thus, from
, we simply enter in the two values for the independent variable x and then calculate the difference between the dependent variable, f, for those given conditions. Remember that a variable is nothing more than a dimension that is allowed to change or take on any value. Thus, from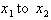 , the change in the independent variable , referred to as
, the change in the independent variable , referred to as 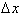 is:
is: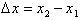
The corresponding change in the dependent variable , referred to as 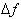 is:
is:
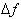 is:
is: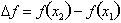
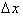 refers to an interval over which we are analyzing the change in the dependent dimension, f.. The second point
refers to an interval over which we are analyzing the change in the dependent dimension, f.. The second point 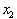 can be written in terms of the first point
can be written in terms of the first point 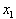 plus the change in the variable,
plus the change in the variable, 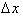
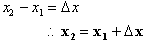
Therefore the change in the dependent variable over an interval from 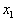 to
to 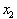 is
is 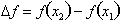 , which can also be written as:
, which can also be written as:
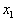 to
to 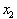 is
is 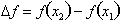 , which can also be written as:
, which can also be written as: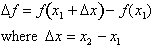
For example the change in the function 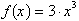 from x=3 to x=5 , where
from x=3 to x=5 , where 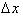 is 5 - 3 = 2 is:
is 5 - 3 = 2 is:
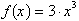 from x=3 to x=5 , where
from x=3 to x=5 , where 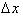 is 5 - 3 = 2 is:
is 5 - 3 = 2 is: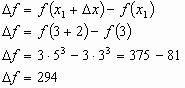
Questions
- Hooke's law states that the force required to stretch a spring is directly proportional to the amount stretched or
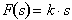 F = Forces = distance stretchedk = spring stiffness constant i.e Force required to stretch spring a unit distanceGraph this function for k=1000 N/m.Calculate the change in force required to stretch the spring from 1m to 4 m.
F = Forces = distance stretchedk = spring stiffness constant i.e Force required to stretch spring a unit distanceGraph this function for k=1000 N/m.Calculate the change in force required to stretch the spring from 1m to 4 m. - The distance a free-falling object covers from an initial dropping point is given by the function:
 d=distanceGiven that acceleration is equal to gravity or
d=distanceGiven that acceleration is equal to gravity or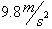 , reduce the three dimensional function to one of two-dimensions for time as a function of distance, d.Graph the function at intervals of
, reduce the three dimensional function to one of two-dimensions for time as a function of distance, d.Graph the function at intervals of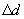 , from d = 0 to d = 100mTabulate the corresponding change in time,
, from d = 0 to d = 100mTabulate the corresponding change in time,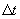 , over each interval.What can you conclude about the change in time covered between d = 10m and d = 20m versus the change in time covered between d = 90 and d = 100m?
, over each interval.What can you conclude about the change in time covered between d = 10m and d = 20m versus the change in time covered between d = 90 and d = 100m?- Solution:
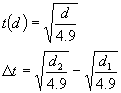 The change from 10m to 20m is 0.59 seconds and from 90m to 100m is 0.23 seconds. So the time is much less from 90m to 100m since the object is travelling a lot faster.
The change from 10m to 20m is 0.59 seconds and from 90m to 100m is 0.23 seconds. So the time is much less from 90m to 100m since the object is travelling a lot faster.
- Solution:
No comments:
Post a Comment